FORM FOUR MATHEMATICS STUDY NOTES TOPIC THREE & FOUR

TOPIC 3: THREE DIMENSIONAL FIGURES

Three Dimensional Figures

Sometimes before you make any purchases you may want to know for example, how much cloth you need to make a pillow cover. What about a cover for your mattress or sofa cushion? How much oil paint do you need to paint your drinking water tank?

What about the amount of cloth for the pocket covers of your radio, curtain, suit, gown, trousers, set of table clothes, etc.

Answers to such questions and of the kind leads you to think more carefully about the size of the surfaces (faces) to be covered or coated on the bodies at work. Perhaps you need to take some measurements on the surfaces.

The knowledge of the surface areas of such bodies will enable you to choose or purchases the required amount without unnecessary wastage so as to minimize purchases costs too.

Three Dimensional Figures

Classify three dimensional figures

Three‐dimensional objects are the solid shapes you see every day, like boxes, balls, coffee cups, and cans.

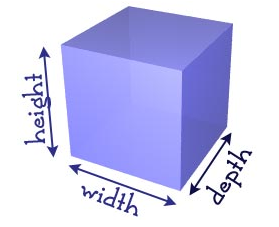
It is called three-dimensional or 3D because there are three dimensions: width, depth and height.

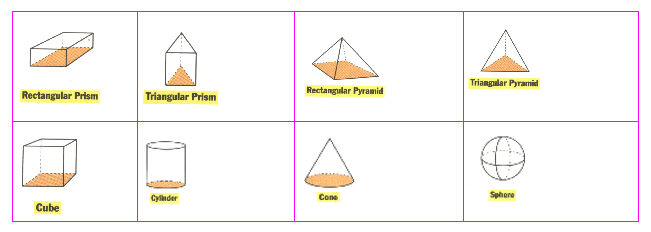
-The following table shows examples of some common three dimensional figures

The Characteristics of Each Class

List the characteristics of each class

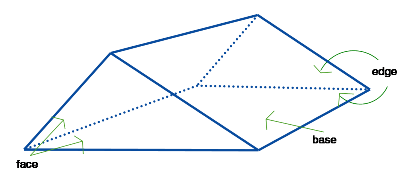
Here are some helpful vocabulary terms for solids:

Base: Is the bottom surface of a solid object.

Edge: Is the intersection of two faces on a solid object. This is a line.

Face: Is a flat side of a 3‐dimensional object.

Prism: Is a solid object with two congruent and parallel faces.

Pyramid: Is a solid object with a polygon for a base and triangles for sides.

Construction of Three Dimensional Figures

Three Dimensional Figures

Construct three dimensional figures

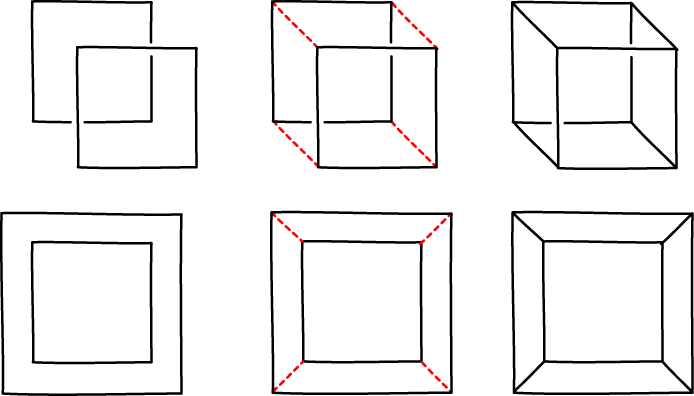
When drawing a three dimensional object it is important to show that it is not a drawing of a flat object. Are usually drawn on a two dimensional plane by making oblique drawings under certain rules as follows:

1. Paralled lines are drawn parallel.
2. Vertical lines are drawn up and down the page.
3. Hidden edges are drawn dotted.
4. Construction lines to guide the eyes are drawn thinly.

Activity 1

Construct three dimensional figures

Sketching Three Dimensional Figures

Three Dimensional Figures

Sketch three dimensional figures

There are several ways of doing the drawing that corresponds to looking at the cube from different angles. The figure shows two ways of doing it.

Properties of Three Dimensional Figures

Identify properties of three dimensional figures

Three dimensional shapes have many attributes such as faces, edges andvertices. The flat surfaces of the 3D shapes are called the faces. The line segment where two faces meet is called an edge. Avertexis a point where 3 edges meet.

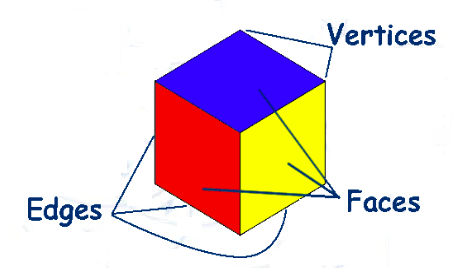
The Angle Between a Line and a Plane

Find the angle between a line and a plane

In finding the angle between the line and a plane in a three dimensional geometry, we use the right angled triangle. Joining the line to define the angle between the line and the plane that provides the least possible angle. Also, projection of one line to another on the plane is mostly used.

Example 1

For the pyramid VABCD with VA=VB=VC=VD=5m, and ABCD a square of side 4cm; find the angle between VA and ABCD.

Solution

Calculated by dropping a perpendicular from V to ABCD. This meets ABCD at X, the centre of the square.

So the projection of VA on ABCD is AX. AC= square root of (AB2 +BC2) =square of 42+42 =square root of 32. AX =1/2square root of32. cos (1/2square root 32)/5=0.5657, so VAX is 55.6.

The Angle Between Two Planes

Calculate the angle between two planes

There are infinite possible lines that could be drawn on planes, making different angles with each other. The angle between planes is the angle between lines within those planes, Must be the lines which are at the middle of the plane for non rectangular planes and any other lines for rectangular planes. Then Right angled triangles are used to find the angles between those planes.

Example 2

Determine theanglebetween the followingplanes:

Surface Area of Three Dimensional Objects

The Formulae for Calculating the Surface Area of Prisms, Cylinder and Pyramids and Cone

Derive the formulae for calculating the surface area of prisms, cylinder and pyramids and cone

Surface Area of a Right Circular Cone

A right circular cone is a cone whose vertex is vertically above the centre of the base of the cone.

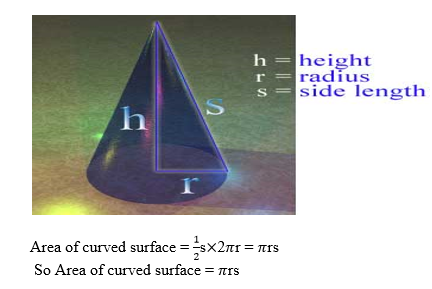
Area of circular base =Πr2 (it is an area of a circle)

Therefore the total surface area of a right circular cone =Πr2 +Πrs = Πr (r + s)

Example 3

Find the total surface of right circular cone whose slant light is 10cm and whose base radius is 8cm.Use Πr(r +s)

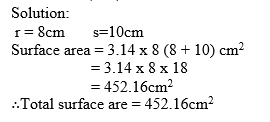
Example 4

Find the total surface area of a cone with diameter 8m and slant height of 10m. Use Π= 3.14

Surface Area of A Right Cylinder

If you want to know the amount of the covering the surface of a blue band margarine can, then you are finding the surface area of a right cylinder. Total surface area of the can is the sum of the areas of the top and bottom. Circular surfaces plus the area of the curved surface,

Now, consider a right cylinder of radius r and height h.

If the cylinder is opened up, the curved surface flattens out to form a rectangle. The length of the rectangle is 2Πr(the circumference of the circular base) and the width is h (the height of the cylinder).

Total surface area of cylinder:

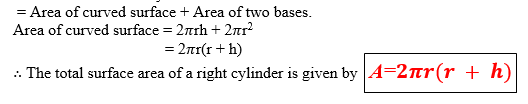
Example 5

Find the total surface area of a cylinder with radius of 3m and height of 10m. Use Π= 3.14

Surface Area of a Right Pyramid

A right pyramid is one in which the slant edges joining the vertex to the corner of the base are equal

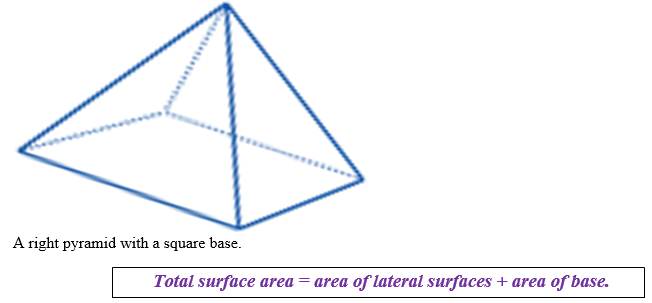
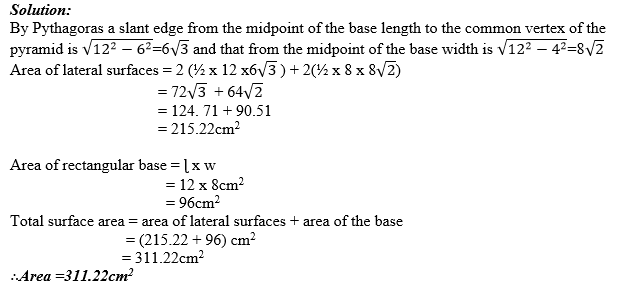
Example 6

A right rectangular pyramid is such that the rectangle is 12cm by 8cm and each slant edge is 12cm. Find the total surface area of the pyramid.

Surface Area of a Right Prism

A full brick or concrete block is an example of a right rectangular prism

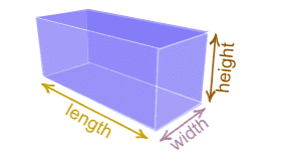
A right prism is a prism in which each of the vertical edges is perpendicular to the plane of the base.

The figure above shows a rectangular right prism in which there are 6 faces though only three of them can be seen easily.

Surface Area = Total or sum of the areas of each face.

Generally for any right prism,

Example 7

The height of a right prism is 4cm and the perimeter of its base is 30cm. Find the area of its lateral surface.

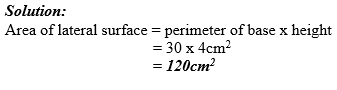
Example 8

Find the total surface area of a rectangular prism 12 by 8 by 6 cm high.

The Formulae to Calculate the Surface Area of Spheres

Apply the formulae to calculate the surface area of spheres

Surface Area of a Sphere

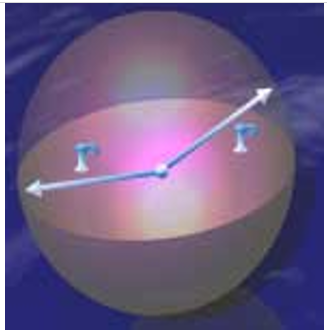
The figure above shows a sphere (ball) with radius “r”

The surface area of a sphere is four times the area of circle with the same radius. The area of a circle is Πr2. Hence, the surface area of sphere is equal to 4Πr2.

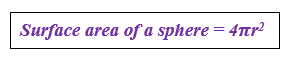
Example 9

Find the surface area of a sphere of radius 5cm. (Π= 3.14)

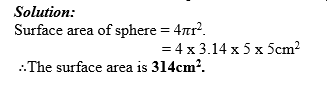
Example 10

Find the surface area of a tennis ball, given that its radius is 3.3cm. UseΠ= 3.14 Express your answer to the nearest tenth.

Exercise 1

Do the exercise to check your understanding. Use π= 3.14 throughout the exercise.

1.The altitude of a rectangular prism is 4cm and the width and lengths of its base are 2cm and 3cm respectively calculate the total surface area of the prism.

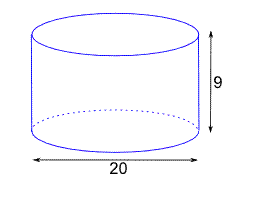
2. The following diagram shows a cylinder of diameter 20 units and height 9 units. What is its curved surface area?

Volume of Three Dimensional Objects

The Formulae for Calculating Volume of Prisms, Cylinders and Pyramids

Derive the formulae for calculating volume of prisms, cylinders and pyramids

VOLUMS OF SOME THREE – DIMENSIONAL FIGURES

We have seen some formulas for calculating the surface areas of some three dimensional figures.

Let us see as well formulas for calculating the volumes of such figures.

-The amount of space that is enclosed by a space figure is called the volume.

The Volume is measured in cubic units, cubic meters (m3), Cubic centimeters (cm3) etc.

When we find (calculate) the volume of a space figure or solid, we are finding the number of

cubic units enclosed by the given spaces figure.

(a) Volume of a Right Prism

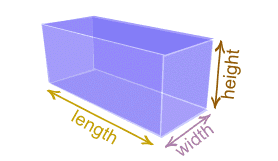
The figure above shows a right rectangular prism. Let h be height, w width and ɭ the length of the prism.

Then the Volume of the prism is given by: V = Base area x height= ɭ×W×h

Generally, volume of any right prism is equal to the product of the area of the base and the height V = Base area x height.

(b) Volume of a Right Cylinder

Consider a right circular cylinder with radius” r “and height h as shown below.

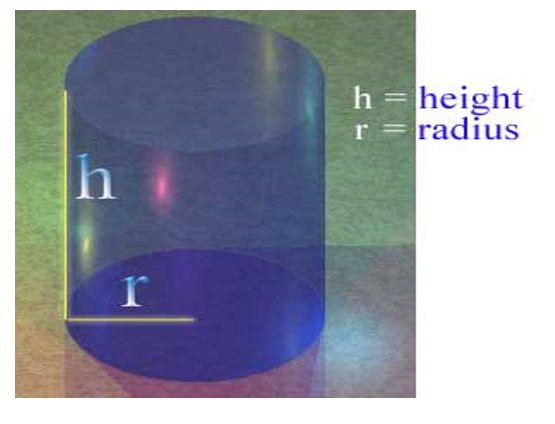
The volume of a right circular cylinder is equal to the product of the area of the base and the height.

If V is volume, A is area of the base and h is the height,

Then Volume = Area of base x height

(c) Volume of a Pyramid

Generally, the volume of a pyramid is one – third the product of its altitude (height) and its base area.

If h is the perpendicular distance from the vertex of the pyramid to its base then,

(d)Volume of a Cone

Consider a cone of radius “r” and altitude h as shown below.

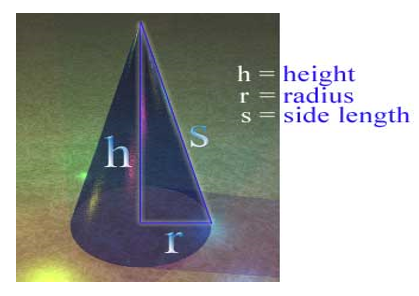
(e) Volume of a Sphere

The figure above shows a sphere of radius r, if the sphere can be put inside a cylinder of the same radius” r”, then the height h = 2r.

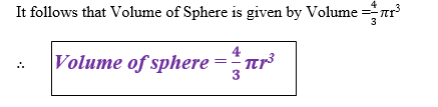
The Formulae to Calculate the Volume of Cylinders, Pyramids and Cones

Apply the formulae to calculate the volume of cylinders, pyramids and cones

Example 11

Find the volume of the prism shown below, given that the dimensions are in meters (m)

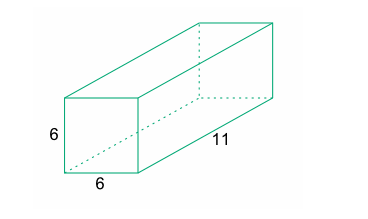
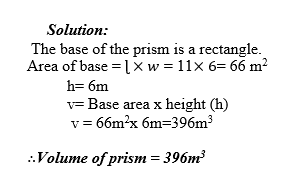
Example 12

Calculate the volume of a rectangular prism whose base is 8cm by 5cm and whose height is 10cm.

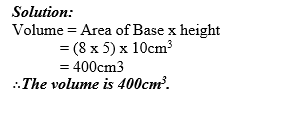
Example 13

Calculate the radius of a right circular cylinder of volume 1570m3 and height 20m. Use π=3.14

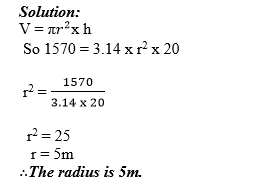
Example 14

A pipe made of metal 1cm thick, has an external (outside) radius of 6cm. Find the volume of metal used in making 4m of pipe. Use π=3.14

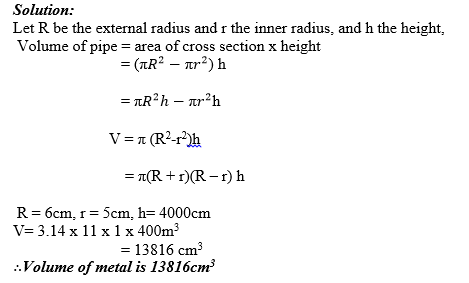
Example 15

Find the volume of a pyramid with rectangular base with length 6m and width 4m if the height of the pyramid is 10m.

Example 16

Calculate the volume of a square pyramid whose altitude is 10cm and length of side of base is 6cm.

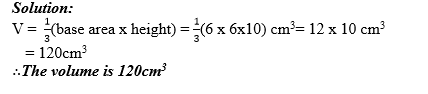
Example 17

Calculate the volume of a cone having base radius 10cm and altitude 12 cm Use π=3.14

Example 18

Find the volume of a sphere whose radius is 10cm. (Take π= 3.14).

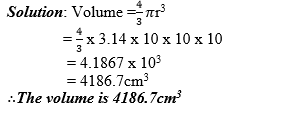
Example 19

The volume of a spherical tank is 268m3. Calculate the radius of the tank. (π= 3.14)

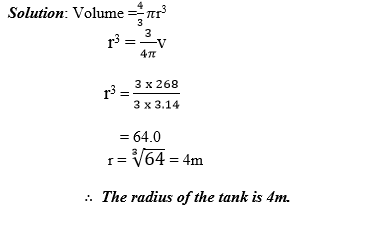
Example 20

Find the volume of rubber in a hollow spherical ball with inner diameter 14cm and outer diameter 16cm. (Take π = 3.14)

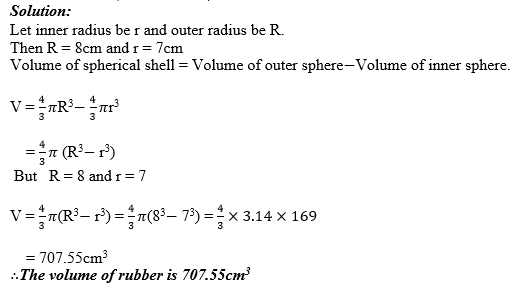
Exercise 2

Answer the following questions and (use π = 3.14)

1. What is the volume of a right prism whose base is a regular hexagon ( n = 6) with a side of the base 4cm long and the height of the prism.

2. Find the volume of a cylinder whose diameter is 28cm and whose height is 12cm.

3. Find the volume of a square pyramid whose height is 24cm and slant edge 25cm each.

4. The slant height of a cone is 20cm and the radius of its base is 12cm. Find its volume in terms of π.

5. The volume of a sphere is 827cm3. Find its radius.

6. A cylinder and sphere have the same volume. If the radius of the sphere is 5cm and radius of the cylinder is 3cm, Calculate height of the cylinder.

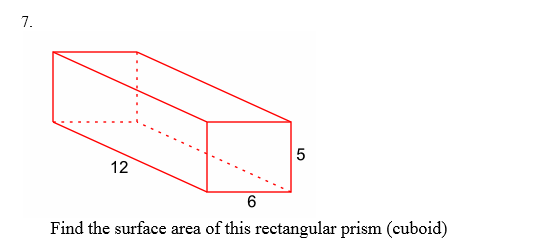
8.The diagram shows a barn. What is the volume of the barn? (The length of the hypotenuse in the right triangle is rounded to the nearest foot.)

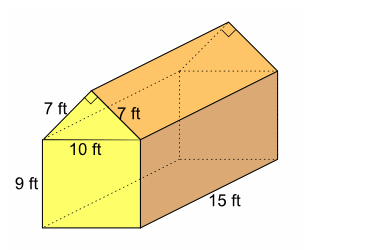
9.What is the volume of this prism?

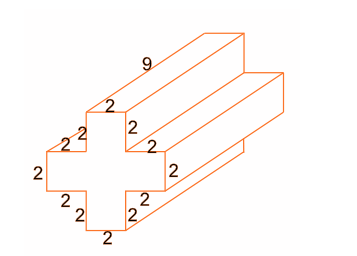
The diagram shows a prism whose cross-section is a right triangle. What is the volume of the prism?

Summary of the topic

Here are the important formulas you have covered under the section on surface areas summarized.

Surface area of:

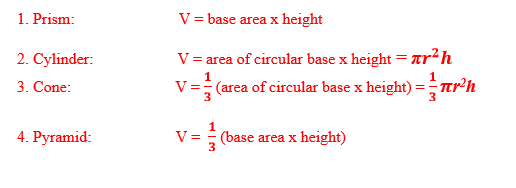
You can now have a summary of the important formulas for calculating volume of some three dimensional figures as follows:-

TOPIC 4: PROBABILITY

Normally we are living in the world full of uncertainties.

For example when two equally strong foot ball teams play a match it is not easy to predicate the outcome of the game. Also for a pregnant woman it is not easy to predict what will be the sex of the born. Under such uncertainties the theory of probability is applied.

Definition; Probability is a branch of mathematics which deals with and shows how to measure the occurrence of events in daily life. Or it can simply be defined as a measure of chances.

Probability of an Events

The Probability of an Even Through Experiments

Determine the probability of an event through experiments

Probability set (s)

Definition: Probability set is the set of all outcomes/results from the experiment being performed.

For example when tossing once a fair coin the expected outcomes are either head(H) or tail(T) to be shown up.

In this case the probability set is

S = {H, T}

Also if a fair die is tossed once what is expected to show up is only one number among the six numbers, that is 1,2,3,4,5,6.

Now the probability set is

S = {1, 2, 3, 4, 5, 6}.

An event (E); An event is a specified outcome from the probability set.

For example a head (H) in the experiment of tossing a fair coin is an event and it is a sub set of the probability set,

Thus, S = {H, T} and E ={H}.

An event may or may not occur. For example if the event that a head occurs in tossing a fair coin once but a tail occurs instead , then the event did not occur and it is dented by E’ which is the complement of E.

So if S = {H, T} and the event E = {H}, then E’ is the event that H does not occur, hence E’ ={T}.

NB: A probability set is also called a sample space

Example 1

1. A dieis tossed once and the results are recorded. Find

1. The probability set (sample space)
2. The event that an even number occurs.
3. The event that an even number does not occur.

Solution;

1. The sample space S ={1,2,3,4,5,6}
2. The event that an even number occurs is E = {2,4,6}.
3. The event that an even number does not occur is E' ={1,3,5}

Example 2

Give the probability set of the experiment of selecting even numbers less than 20.

Solution

S = {2, 4, 6, 8, 10, 12, 14, 16, 18}.

Example 3

Give the probability set of not selecting an even number from a set of counting numbers less than 9.

Solution

S= {1, 2, 3, 4, 5, 6, 7, 8}

E = {2, 4, 6, 8}

So E' = {1, 3, 5, 7}

where E is the event of selecting an even number and E' is the event of not selecting even number less than 9.

Exercise 1

1. Write theprobability set of each of the following experiments:

1. A die is tossed and the face showing up is read.
2. A friend is asked for the month of his birth.
3. The sex of a human being is asked.
4. A card is drawn from a box containing five cards bearing the numerals 2,4,6,8 and 10.

2. Write inset notation the elements of the following events:

1. A fair die is rolled and the number obtained is greater or equal to 5.
2. A prime number between 20 and 40 is chosen.

3. Write inset notation the elements of the event of not choosing an even number between 25 and 55

Experimental Results in Relation to Real Life Occurrences

Interpret experimental results in relation to real life occurrences

For example when tossing once a fair coin the expected outcomes are either head(H) or tail(T) to be shown up.

In this case the probability set is

S = {H, T}

Also if a fair die is tossed once what is expected to show up is only one number among the six numbers, that is 1,2,3,4,5,6.

Now the probability set is

S = {1, 2, 3, 4, 5, 6}.

The Formula for Finding the Probability of an Event

Write the formula for finding the probability of an event

Probability of an event:

Definition: The probability of an event is the ratio between the number of times the event has occurred to the total number of experiments that have been done.

If P(E) Is the probability of the event E, then

Also the probability found by experimenting is referred to as experimental probability.

The Formula to Calculate the Probability of an Event

Apply the formula to calculate the probability of an event

Example 4

A drawing pin was tossed 1000 times. The number of tosses where the pin fell flat was 563. Calculate the probability that when such a pin is tossed, it will fall flat.

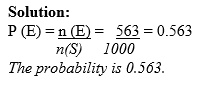
Example 5

5% of torch bulbs manufactured by a certain factory were defective. What is the probability that when a bulb from that factory is tested it will be defective?

Solution:

P(E) = 5% = 5/100 = 0.05

Note that the probability of an event is defined under the condition that every outcome has an equal chance of occurring as other outcomes. Here we say the outcomes are equally likely or equiprobable.

Words like random selection, fair die and a fair coin are used mean that the choice is impartial (unbiased)

Example 6

A piece of chalk is picked from a box containing 5 identical pieces two of which are red and the remaining are white. Find the probability that the piece of chalk picked is red

Example 7

Find the probability that a ling appears in a drawing a single card from an ordinary deck of 52 cards.

Example 8

What is the probability of not getting an even number when a fair die is tossed?

Example 9

What is the probability of selecting a green ball from the box containing red and green balls if the probability of selecting red ball is 1/4?

Example 10

When tossing a die what is the probability of getting a number greater or equal to 1?

Exercise 2

For practice.

1. Find the probability of choosing a number divisible by 2 from a set of numbers between 20 and 45.
2. The total number of red and white pieces of chalk that are contained in a box is 20. How many pieces of white chalk are in the box if the probability of choosing a red piece of chalk is 2/5, given that the pieces are identical?
3. What is the probability that a month selected at random from the twelve months of the year will have 31 days?
4. A survey conducted at certain maternity ward showed that 60% of children born were female. What is the probability that Moses’ child, who was born in that ward is a male?
5. A die was tossed 100 times, the six numbers with their frequency of occurrence were recorded in the following table:

Combined Events

Experiments of Two Combined Events

Perform experiments of two combined events

If two or more simple events may occur or take place at the same time then the events are combined events.

For instance when the experiment of tossing two coins at the same time is done, then the event of interest can’t simply be determined.

Let E = {Obtaining two heads}

In this case there are two simple events which are obtaining the head on the first coin and obtaining the head on the second coin.

So E1 = {Obtaining the head on the first coin}

E2 = {Obtaining the head on the second coin}

Drawing a Tree Diagram of Combined Events

Draw a tree diagram of combined events

The event E can be found by using what is referred to as a tree diagram.

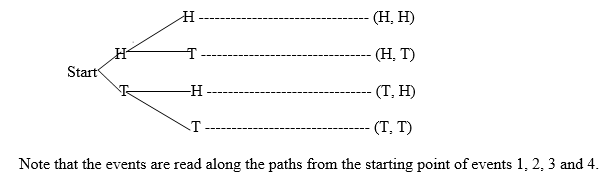
Therefore, S = {(H, H), (H, T), (T, H), (T, T)} is the sample space.

Example 11

A die and Coin are tossed together. Draw a tree diagram to find the Sample space and hence determine the probability that a head and a number less than 3 occurs.

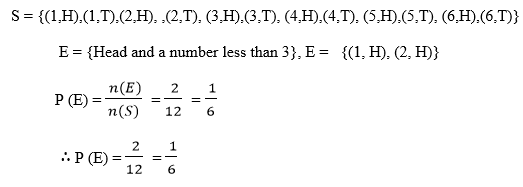
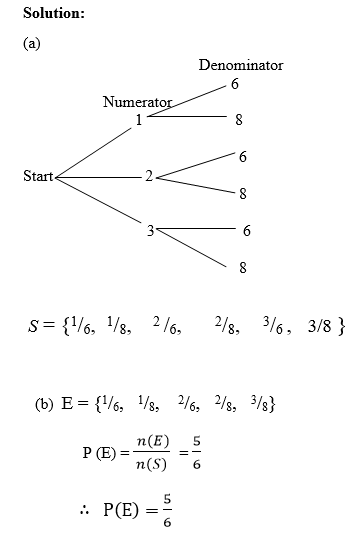
Example 12

A fraction is written by selecting the numerator from the digits 1, 2, 3 and the denominator from the digits 6, 8.

1. Draw a tree diagram to find the sample space of this experiment.
2. Find the probability that a the fraction written is less than ½

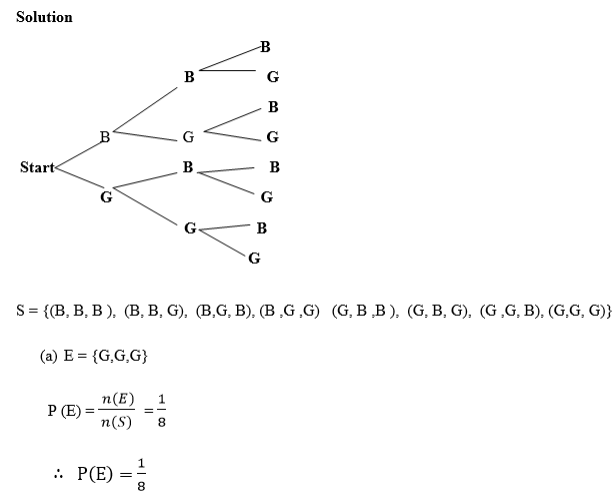
Example 13

In a family of 3 children what is the probability that

1. All are girls
2. At least two are boys

Example 14

Three coins are tossed simultaneously. Find the probability that

1. 3 heads appear
2. 2 tails and one head appear

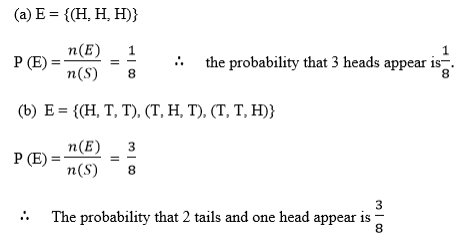
Exercise 3

1. If two digitsnumeral is written choosing ten’s digits from the set {1, 2, 3, 4,} and the unit’s digit from {5,6} what is the probability that a number greater than 20 will appear?

2. A pair of dice istossed. Find the probability that the sum of the two numbers obtained is

1. At least 8
2. at most 1
3. Exactly 6

3. In a familywith two children, what is the probability that:

1. Both are boys
2. At least one is a boy

4. A die and twocoins are tossed at the same time find the probability that

1. A number 6 and two heads will appear.
2. A number less than 4, a head and tail will appear.
3. A number multiple of 2 and two tails will appear.

The Probability of Two Combined Events using the Formula

Find the probability of two combined events using the formula

Mutually Exclusive Events;

Two or more events are said to be mutually exclusive if the occurrence of one event hinders the occurrence of the other. This means that for mutually exclusive events, only one event may occur at a time, e.g., it is impossible for two numbers say 1 and 6 on a single die to show up for one tossing.

Therefore if A and B are two events, then the probability of A or B is given by

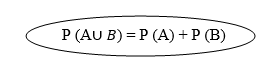
Example 15

If in a class there are 34 students instead of 35 and Issa, anna, Eliza and Juma apply for the one chance remaining what is the probability that either Anna or Juma will be chosen?

Example 16

Find the probability that an even or an odd number which is greater than 1 occurs when a die is tossed once.

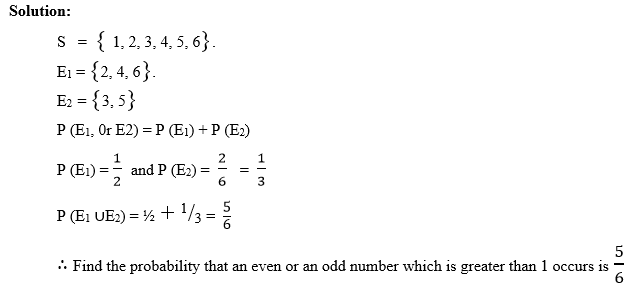
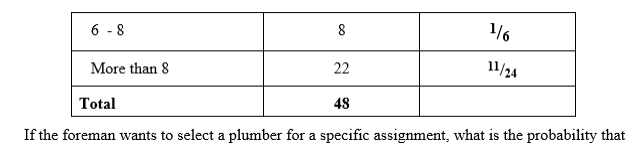
Example 17

The following table shows years of experience for plumbers in a builders company.

Independent Events

Definition: Two or events are said to be independent events if the occurance of one event does not affect the occurrence of other event(s)

For example when a die and a coin are tossed together, the occurrence of a tail on the coin does not hinder the occurrence of the number 5 on the die.

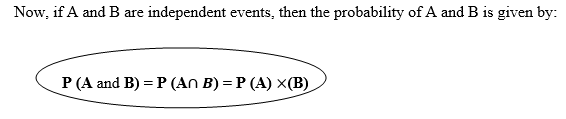
Example 18

A die and coin are tossed. Find the probability that a number greater than 4 appears on the die and a tail appears on the coin:

Example 19

A box contains 9 oranges, 7 mangoes and 2 lemons. A fruit is drawn from the box and then replaced. Another draw is made. What is the probability that both fruits drawn are mangoes.

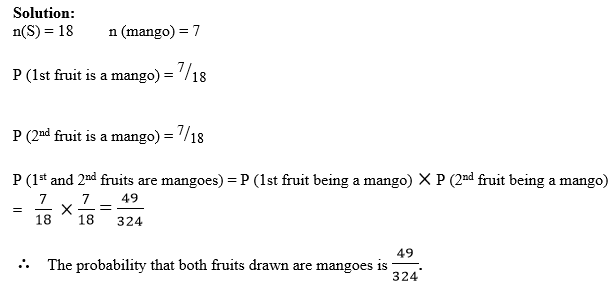
Example 20

The probability that a man and his wife will be alive for 50 years are 3/10 and 1/3 respectively. Find the probability that

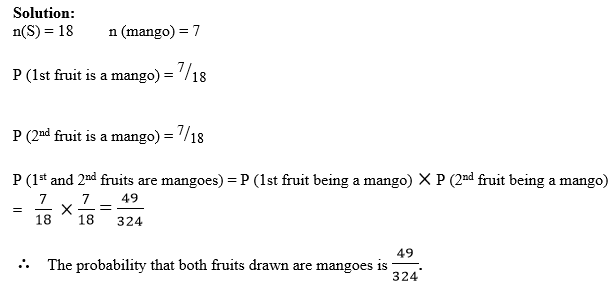
Exercise 4

Self test.

1. A coin is tossed and a card is drawn from an ordinary pack of 52cards.Find the probability that an ace is drawn and a head is obtained on the coin (There 4 aces in a pack of cards)
2. Two numbers are selected from the integers 1 to 11 inclusively, repeation being allowed. Find the probability that (a) Both prime (b) Both are powers of 2

3. In the village,the probability that a man selected at random on a Sunday morning is carrying more than is 0.7. Find the probability that;

1. Two men selected at random on a Sunday morning is carrying more than 30kg
2. Three men selected at random are all carrying more than 30kg

4. A letter is chosenfrom the word “random” What is the probability that it is an n or d?

5. (a) What does itmean by saying that the probability of an event is (i) 0 (ii) 1 (b) Give two examples of impossible of events.

The Knowledge of Probability to Determine the Occurrence of Events in Real Life Situation

Apply the knowledge of probability to determine the occurrence of events in real life situation

Probability is an area of mathematics which we use all the time in daily life – and usually without thinking about it. While many aspects are very intuitive, probabilities may be different for different people. I might estimate that the chance of rain is 70%, while a meteorologist with detailed weather data might say the chance of rain is 64.2%.

Activity 1

Apply the knowledge of probability to determine the occurrence of events in real life situation